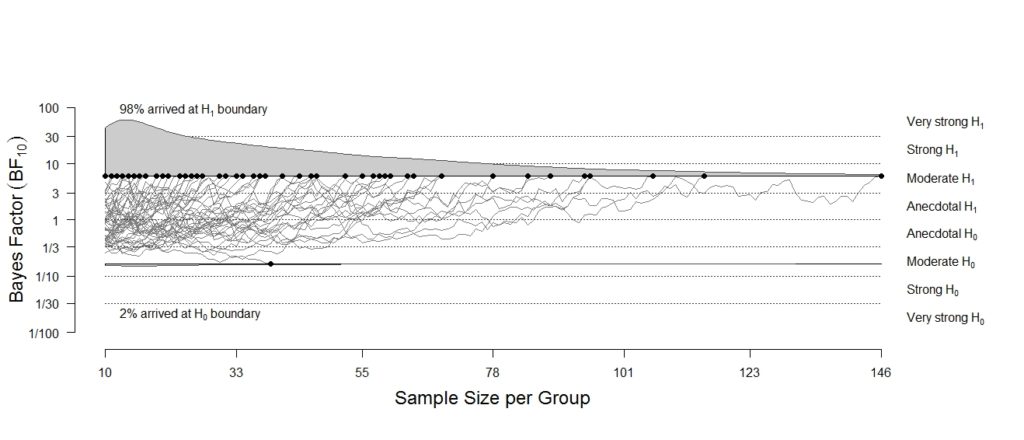
Popular Misconceptions About Bayesian Inference: Introduction to a Series of Blog Posts
“By seeking and blundering we learn.” – Johann Wolfgang von Goethe Bayesian methods have never been more popular than they are today. In the field of statistics, Bayesian procedures are mainstream, and have been so for at least two decades. Applied fields such as psychology, medicine, economy, and biology are slow to catch up, but in general researchers now view…
read more