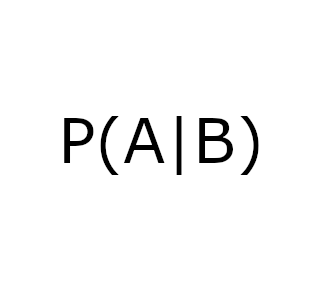Book Review: “The Seven Deadly Sins of Psychology”

This book review is a translated and slightly adjusted version of one that is currently in press for “De Psycholoog”. The review was inspired by the recent Dutch translation De 7 Doodzonden van de Psychologie (see references below). In his inaugural address on September 11th 2001, Diederik Stapel made a bold claim about the prestige and accomplishments of the field…
read more